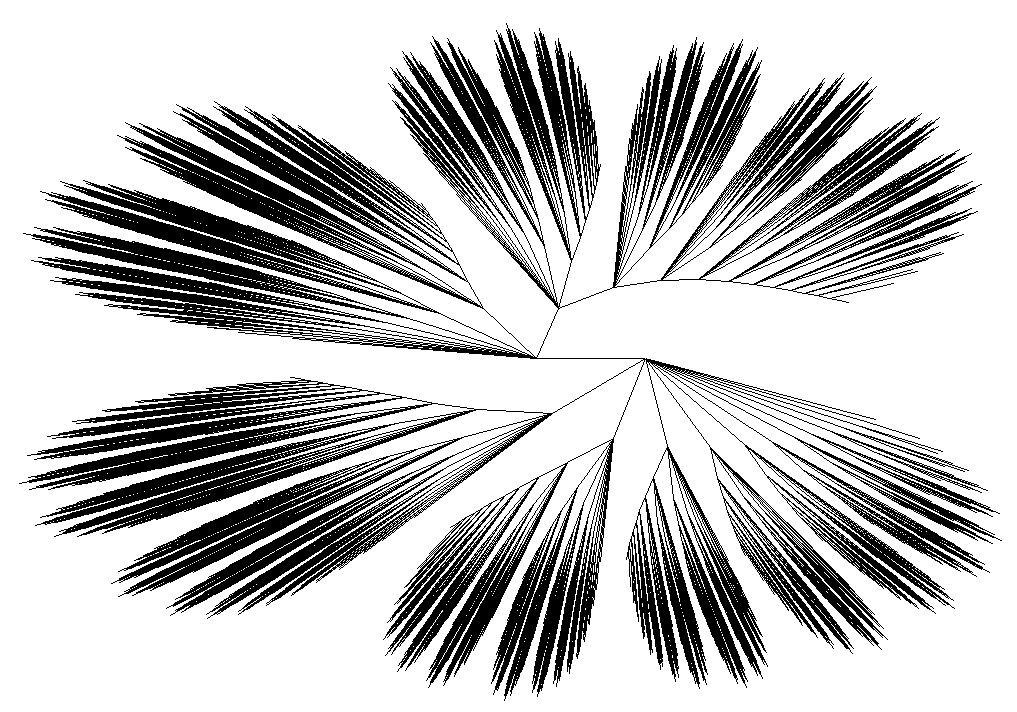
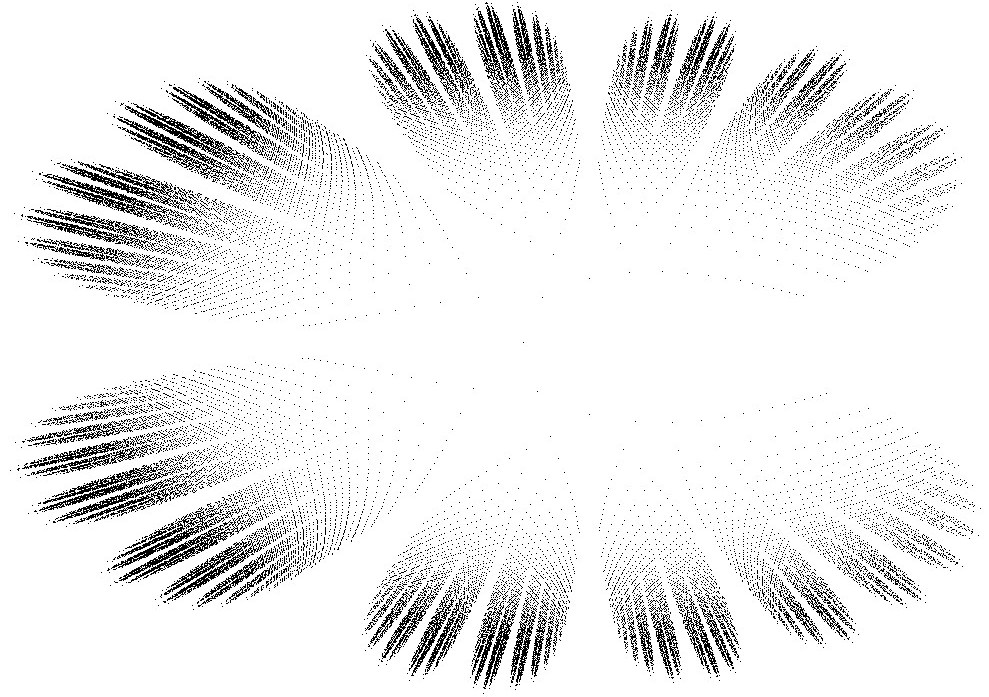
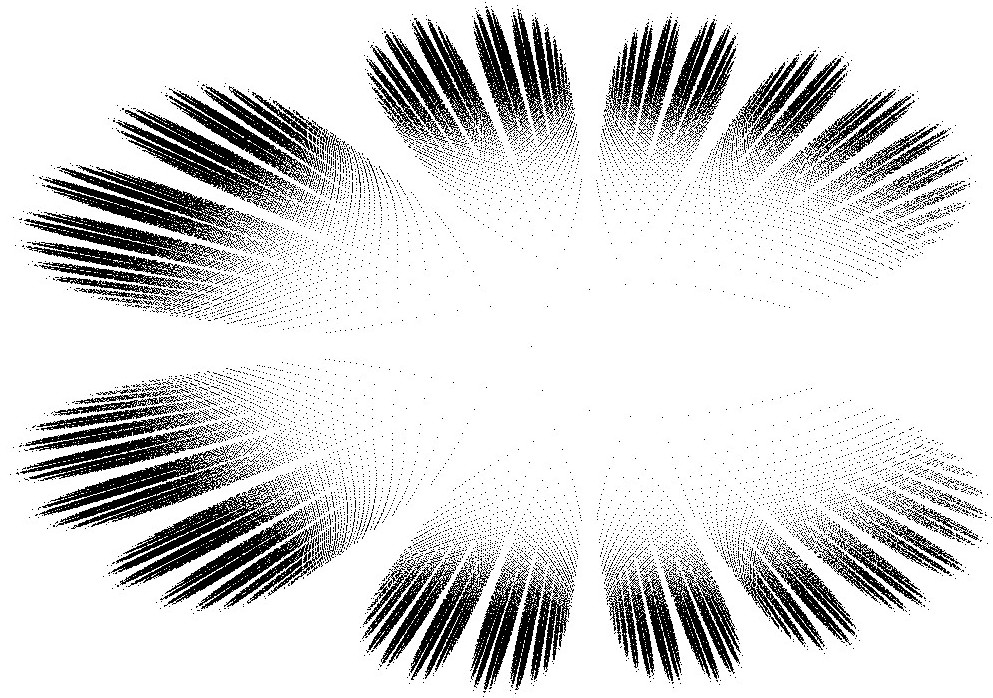
These are based on Farey sequences, (well, more properly, the left half 
of the Stern-Brocot tree) drawn in the positive x-y plane between 
and 
, and expanded (i.e. by 8) anti-clockwise around the origin so that the fraction value ![\quad[\frac{0}{1}\ldots\frac{y}{x}\ldots\frac{1}{1}]\quad](https://www.adamponting.com/wp-content/ql-cache/quicklatex.com-79fbbb8bb841034f8144c3b02f7913fe_l3.png "Rendered by QuickLaTeX.com")
is represented as ![\quad[0\ldots\theta\ldots2\pi]\quad](https://www.adamponting.com/wp-content/ql-cache/quicklatex.com-2733470b315c63d848cbf448ea9ab1cc_l3.png "Rendered by QuickLaTeX.com")
radians, i.e. in ![\quad[0^{\circ}\ldots360^{\circ}]](https://www.adamponting.com/wp-content/ql-cache/quicklatex.com-8a4d758e888c44b7e8c2f6c390d4f544_l3.png "Rendered by QuickLaTeX.com")
(from 3 o’clock anticlockwise around to 3, in clock-speak).
Each new row of the Stern-Brocot tree is made from the previous one by putting a new fraction between each pair, adding together the numerators and denominators (i.e. top and bottom) to get the new values, i.e.
![\[\{\frac{0}{1},\frac{1}{1}\}\quad \{\frac{0}{1},\frac{1}{2},\frac{1}{1}\}\quad \{\frac{0}{1},\frac{1}{3},\frac{1}{2},\frac{2}{3},\frac{1}{1}\}\quad \{\frac{0}{1},\frac{1}{4},\frac{1}{3},\frac{2}{5},\frac{1}{2},\frac{3}{5},\frac{2}{3},\frac{3}{4},\frac{1}{1}\}\]](https://www.adamponting.com/wp-content/ql-cache/quicklatex.com-c2a12faea324415368ff4d2e4050e909_l3.png "Rendered by QuickLaTeX.com")
Continuing on in this way, the set will contain every fraction between 0 and 1, and all in lowest terms! (i.e. never 
or 
etc)
It has many amazing properties and applications, but strangely was not described until the 19th C.
The big gap on the middle left hand side is the region of 
, and then gaps clockwise around from there, each one smaller, are 
, 
, 
, 
, 
, etc. The ones on the underside are, from left, 
, 
, 
, 
, 
etc.
