I made a picture of the frequencies produced in going up in octaves, in fifths (perfect, not the imperfect ‘perfect’ fifth of equal temperament), and in pure major thirds (2x, 3/2x and 5/4x frequencies), up to 12 octaves higher, to find other close coincidences besides the one that’s the basis of western music, i.e.
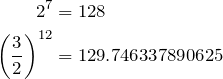
i.e. if you go up in 5ths – C-G-D-A-E etc, you come back to a C again 7 octaves up and 12 notes later.
and I found
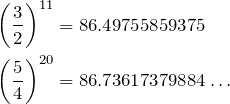
i.e. if you go up in major 3rds, you come back to the ‘same note’ 11 5ths up and 20 notes later.
which is much closer agreement than the Pythagorean comma. It is a scale built from thirds and fifths (x5/4 and x3/2), not the octaves and fifths (x2 and x3/2) we know and love. I guess it’s the basis of the Bohlen-Pierce scale, with its tritaves etc? Apparently Wendy Carlos found it with a computer search a few years ago, called it the gamma scale, and used it on one of her records.
The only other close calls up to 7 octaves seem to be:
![]()
![]() and
and ![]()
![]() and
and ![]()